Description of the Method

Analytic Hierarchy Process (AHP) is a form of  multi-criteria analysis (see Policy Briefing Note 6) that is used to analyse complex decisions where multiple perspectives need to be considered. It was developed by Saaty (1980) to help decisionmakers find the option that best suits their goal and understanding of the ‘problem’, while taking into consideration factors that cannot be quantified.

AHP is very flexible and can be adapted to different needs and contexts. Criteria (or attributes) and sub-criteria can be decided in advance by the expert or through a participatory process with stakeholders to increase transparency, dialogue and ownership of the process and outcome. There is no upper limit to the number of criteria or sub-criteria, except for the time that is required to do the comparison.

Criteria can be both tangible and intangible and defining them can involve as many participants as required. The number of alternatives to evaluate can also vary, though they should be as mutually exclusive as possible. The types of decision situations in which the AHP can be applied include choices, ranking, prioritisation, resource allocation and conflict resolution and clearly these have relevance in many areas of climate adaptation.


A series of steps are involved in undertaking the method (Saaty, 1980: 2005: 2008):

1. Define the ‘problem’ or adaptation challenge, i.e. the need and purpose of the decision (goal), listing the alternatives to evaluate (e.g. adaptation options), setting-up the criteria and sub-criteria (attributes) by which to evaluate the alternatives (or adaptation options) and identifying the stakeholders and groups to involve in the process.
2. Structure the issue, including the decision hierarchy, and identify the top-level criteria, the intermediate criteria, and the set of options.
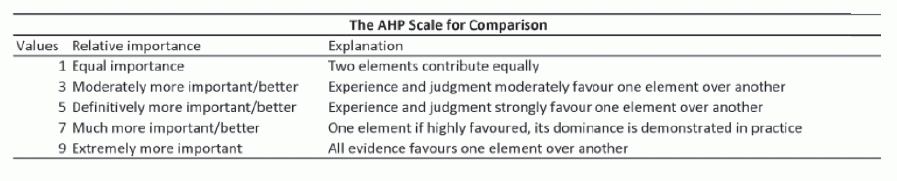
3. Undertake the pairwise comparison. This compares the elements to one another, two at a time, with respect to their impact/ importance on an element above them in the hierarchy. This uses numerical values (e.g. as in the scale below) to conduct the pairwise comparisons, constructing a set of pairwise comparison matrices. Several matrices are produced to compare the alternatives (e.g. adaptation options) with respect to each criteria, and the criteria with respect to the goal.
4. Calculate relative priorities. This can be done using a spreadsheet, or software, such as ExpertChoice (http://expertchoice.com/). The values in Step 3 are processed to obtain numerical priorities or weights for the criteria and alternatives. Depending on the problem at hand, a priority or weight can refer to importance, preference or likelihood.
5. Aggregate priorities. The final step is to aggregate relative priorities to produce overall priorities (final evaluation metrics) which sum to 1.0.

• Section overview
• Key Messages
• Introduction
• Description of the Method
• The Application to Adaptation
• Strengths and Weaknesses
• Case Studies
• Discussion and Applicability
• References

---

    download this briefing note

---