Let us return to system (5) and analyse it
graphically. We can get the value ![]() as a parametric
function of
as a parametric
function of ![]() from the first equation of (5) (figure
1). It is obvious that the set of admissible
from the first equation of (5) (figure
1). It is obvious that the set of admissible ![]() will
belong to the interval
will
belong to the interval ![]() where
where ![]() is a solution of
the equation
is a solution of
the equation ![]() and
and ![]() . On the other hand,
. On the other hand, ![]() , or, since
, or, since ![]() then
then ![]() (
(![]() is a
solution of the equation
is a
solution of the equation ![]() ) and
) and ![]() . Using
the analogous considerations for the second equation of
(5) (figure 2) we get
. Using
the analogous considerations for the second equation of
(5) (figure 2) we get ![]() and
and
![]() . At last,
we have
. At last,
we have
![]()

Figure 1: Graphical solution of (5): ![]() or
or ![]() .
.
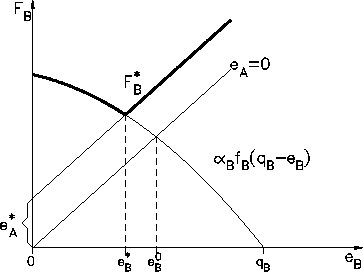
Figure 3: Extreme case when the actor B is a super-altruist (![]() ).
).
Let us imagine the situation when
![]()
From the first equation of (5) and the inequality (7)
we get the following chain of inequalities:
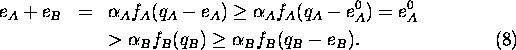
From (8) and the definition of the utility function ![]() follows that
follows that
![]()
i.e., the utility function of the second actor is
determined only by the common interest. A graphic
illustration of this statement is shown in figure 2. From
the altruistic viewpoint the emission must be reduced to
zero (![]() ). Simultaneously the utility function of the
first actor,
). Simultaneously the utility function of the
first actor, ![]() , and his equilibrium
strategy,
, and his equilibrium
strategy, ![]() , depends only on his behaviour: he minimises
the own losses knowing that his altruistic partner will
always reduce his emission to zero. In other words,
the first actor can be considered as an egoist, who does
not take into account the interest of another partner.
, depends only on his behaviour: he minimises
the own losses knowing that his altruistic partner will
always reduce his emission to zero. In other words,
the first actor can be considered as an egoist, who does
not take into account the interest of another partner.
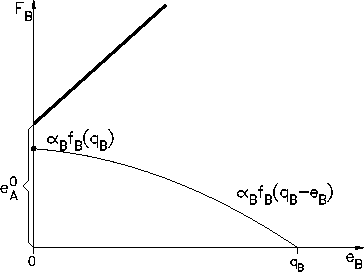
Figure: Extreme case when the actor B is a super-altruist (![]() ).
).
There are two marginal ways to realise the inequality
(7): either the emission ![]() is very small so that the
expenditures for its reduction are relatively small, too,
or the coefficient of egoism
is very small so that the
expenditures for its reduction are relatively small, too,
or the coefficient of egoism ![]() is very small. In order
to realise this we have to suggest that either the actor
B is a very small country with low total emission or
he is a typical rural country with agricultural economy,
or he is a ``super-altruist''.
is very small. In order
to realise this we have to suggest that either the actor
B is a very small country with low total emission or
he is a typical rural country with agricultural economy,
or he is a ``super-altruist''.
Such a sort of discrimination among actors is a
typical consequence of the non-negativity of emission, so
that ![]() is necessary. But if we neglect such a constraint,
then the equations (5) will have a solution with
is necessary. But if we neglect such a constraint,
then the equations (5) will have a solution with ![]() also in the case (7). Note that if
also in the case (7). Note that if ![]() then
then ![]() (and, on the contrary, if
(and, on the contrary, if ![]() then
then ![]() ). In other
words, extending the set of admissible strategies, we
provide the existence of an equilibrium strategy in any
case. How can we interpret a ``negative emission''? For
instance, this may be the formation of some carbon sink
on the territory of the country by means of reforestation.
). In other
words, extending the set of admissible strategies, we
provide the existence of an equilibrium strategy in any
case. How can we interpret a ``negative emission''? For
instance, this may be the formation of some carbon sink
on the territory of the country by means of reforestation.