and 
The function 
, as a function of N, will have the following form
(see Fig. 5).
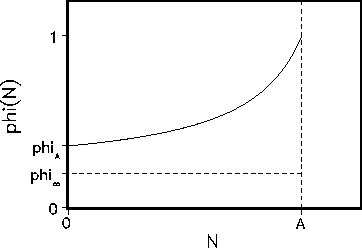
Figure 5: The function 
(considering the greenhouse effect in the model) as a function of N. The value
, corresponding to zero value of N, is determined by
the value A. Since we shall consider this function as a function of N:
, then 
.
Analogously to the Daisyworld model by Watson and Lovelock, the vegetation cover changes the albedo 
of the planet. We suppose that the surface albedo decreases with an increase of vegetation. In Fig. 6 the functional form of 
is plotted.
Figure 6: The function considering the solar irradiance: 
, 
.
In order to detect the equilibria we shall need the function 
. It is obvious that 
, where
, and
. If 
at some point 
, where
the expression
is defined as
then 
has a maximum at 
(see
Fig. 7).
Figure 7: The function 
; at the point one gets 
, or
.
If 
then is a monotonous increasing function, and if 
is a monotonous decreasing one.