Description

The essential theoretical foundations of CBA are: benefits are defined as increases in human well-being (utility) and costs are defined as reductions in human wellbeing. For a project or policy to be justified on cost-benefit grounds, its social benefits must exceed its social costs. Hence CBA is also called societal CBA, if cost and benefits are assessed from the perspective of society as a whole. The initial step of CBA is to determine whose costs and benefits and the time horizon over which costs and benefits are counted. Second, CBA has to consider the time-preference through the process of discounting because individuals have preferences for when they receive benefits or suffer costs. Costs and benefits are rarely known with certainty so that risk (with probabilistic outcomes) and uncertainty (when no probabilities are known) also have to be taken into account. The decision rule for comparing costs and benefits is the net benefits criterion. A standard CBA involves calculating the present values of the social costs and benefits of a project or an adaptation option (PVC and PVB) and their difference (NPV) or their ratio (B/C), i.e.



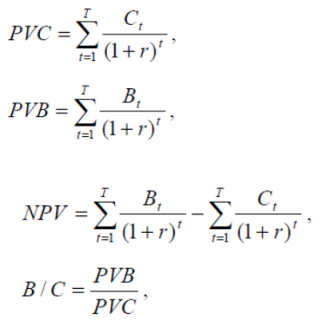
where Ct is the social costs and Bt is the social benefits of the project in the year t, T is the life time of the project and r is the discount rate.

If NPV ≤ 0 or B/C ≤ 1, then the project adds no net welfare to society and the project should not be pursued because society would not be made better-off, if all benefits of adaptations can be quantified and monetised. If NPV > 0 or B/C > 1, then the project adds welfare to society.

Toolbox tags

This toolbox entry has been labelled with the following tags:

Sector:		independent
Spatial scale:		independent
Temporal focus:		independent
Onset:		independent
Role in decision process:		prescriptive
Level of skills required:
Data requirements:
Adaptation tasks:		Expected outcomes: utility, welfare, effect etc. (e.g. CEA, CBA)

Strengths and Weaknesses

All projects with a positive NPV should, in principle, be undertaken because they add to the welfare of society, but budget constraints prevent this from happening. A project with a positive NPV may not proceed because an alternative project has a higher NPV. When there are a number of projects and programs available to decision makers with a limited budget, it is necessary to rank projects.

However, for adaptation, the use of standard CBA can be limited, primarily because of the partial availability of data on the costs and benefits of adaptation options. There are also other reasons, amongst which may be the distribution of impacts, especially on the particularly vulnerable, although these can be accounted for through the inclusion of distributional weights in analysis. Further, CBA fails to account for those costs and benefits that cannot be reflected in monetary terms, particularly such as ecological impacts, as well as concerns that influence welfare, such as peace and security. Subject to this qualification, it can be applied to decisions in some sectors for certain types of adaptation options (e.g. technical measures for flood prevention), or in sectors where there is a major private sector involvement (UNFCCC, 2010).

Applicability

Richards and Nicholls (2009) applied cost-benefit analysis to some adaptation options (raising dykes and beach nourishment) in the costing and adaptation module of the DIVA model for assessing impact and vulnerability of the coastal systems in Europe and determining the level of adaptation. The specific adaptation assessment options focused on reducing flood risk through the construction and increase in height of flood defence dikes and reducing beach erosion through placing of additional sand onto exiting beach areas, which are considered public-funded and the coast is seen as a public good, and hence all adaptation costs are considered to be public investments. The costs include the sand costs for beach nourishment, the construction costs for national dike, and other costs related to increased river flooding in the lower reaches of rivers subject to the influence of sea level and the construction of river dikes. In the DIVA model, it is assumed that the adaptations take place where is economically optimum, as determined by cost-benefit analysis.

Other examples of CBA includes applications to sea level rise as reported in Agrawala and Fankhauser (2008), to fresh water systems (Callaway et al, 2007) and to the agricultural sector (e.g. Rosenzweig and Tubiello, 2007).

Accessibility

CBA is not a proprietary tool or software package, and is discussed in the toolbox as the operationalization of the method. Thus, only limitation to access is the knowledge required to perform CBA.

Further information on CBA can be found via the in-depth in the Adaptation Task Navigator.

Further Reading and References

UNFCCC, 2010. Potential costs and benefits of adaptation options: a review of existing literature.

Richards J.A., R. J. Nicholls. 2009. Impacts of climate change in costal systems in Europe. PESETA-coastal systems study. EUR 24130 EN, JRC, European Communities.

Rosenzweig C and Tubiello F. 2007. Metrics for Assessing the Economic Benefits o fClimate Change Policies in Agriculture. Organisation for Economic Co-operation and Development: Paris.

Callaway JM, Louw DB, Nkomo JC, Hellmuth ME and Sparks DA. 2007. The Berg River Dynamic Spatial Equilibrium Model: A New Tool for Assessing the Benefits and Costs of Alternatives for Coping With Water Demand Growth, Climate Variability, and Climate Change in the Western Cape. AIACC Working Paper No. 31. Available at http://www.aiaccproject.org/working_papers/Working%20Papers/AIACC_WP31_Callaway.pdf.

Agrawala, S. and Fankhauser, S. (Eds.) (2008) Economic Aspects of Adaptation to Climate Change: Costs, Benefits and Policy Instruments. OECD

Toolbox category

This detail page belongs to toolbox category
CBA

---

weADAPT case studies identified for this toolbox entry: